Intro

Which works better for a given function – a Taylor expansion or a Fourier Expansion?  This post explores the pros and cons of each, using specific examples.

Examples of Taylor and Fourier Series Expansions

1. Polynomial Function: 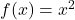f(x) = x^2

Taylor Series Expansion: x^2 = x^2

Fourier Series Expansion: x^2 = \frac{\pi^2}{3} + \sum_{n=1}^{\infty} \frac{4(-1)^n}{n^2} \cos(nx)

Best Fit: Taylor series

2. Trigonometric Function: 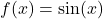f(x) = \sin(x)

Taylor Series Expansion: \sin(x) = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \dots

Fourier Series Expansion: \sin(x) = \sum_{n=1, \text{odd}}^{\infty} \frac{4}{n\pi} \sin(nx)

Best Fit: Fourier series

3. Exponential Function: 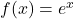f(x) = e^x

Taylor Series Expansion: e^x = 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \dots

Fourier Series Expansion: Not practical

Best Fit: Taylor series

4. Piecewise Function: f(x) = |x| on [-\pi, \pi]

Taylor Series Expansion: Not possible

Fourier Series Expansion: |x| = \frac{\pi}{2} - \sum_{n=1, \text{odd}}^{\infty} \frac{4}{n^2\pi} \cos(nx)

Best Fit: Fourier series

5. Periodic Step Function: f(x) = \text{sgn}(\sin x)

Taylor Series Expansion: Not possible

Fourier Series Expansion: f(x) = \frac{4}{\pi} \sum_{n=1, \text{odd}}^{\infty} \frac{1}{n} \sin(nx)

Best Fit: Fourier series

Comparison Table

Function Taylor Series Fourier Series Best Fit
x^2 Good (converges well) Works if periodic but inefficient Taylor series
\sin(x) Good for small x Best for periodic representation Fourier series
e^x Excellent (globally convergent) Poor (unless forced periodicity) Taylor series
|x| Not possible Works well (some Gibbs effect) Fourier series
\text{sgn}(\sin x) Not possible Best option (Gibbs phenomenon) Fourier series